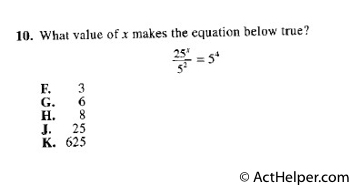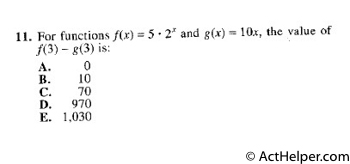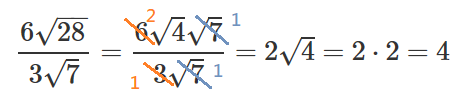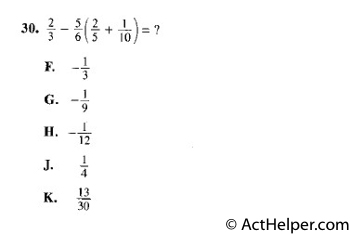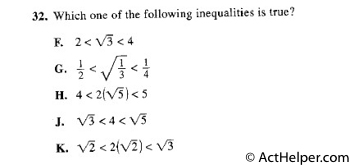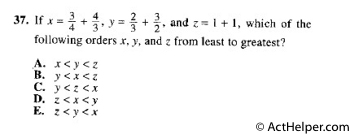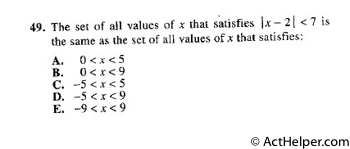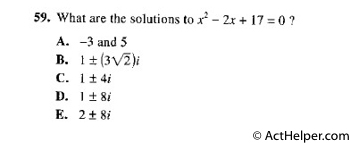Choice C
$$P(Not\ white)$$ $$=1-P(White)$$ $$=1-\frac{12}{21}$$ $$=\frac{9}{21}$$ $$=\frac{3}{7}$$
Choice A
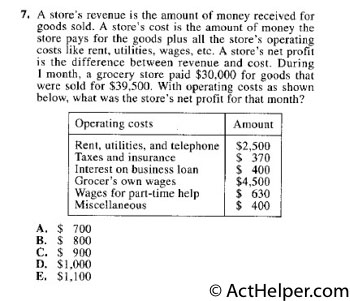
$$ $39500-$30000=$9500 $$ $$ $2500+$370+$400+$4500+$630+$400=$8800 $$ $$ $9500-$8800=$700 $$
Choice A
$$5-\frac{3}{4}-2\frac{1}{2}$$ $$=\frac{20}{4}-\frac{3}{4}-\frac{10}{4}$$ $$=\frac{7}{4}$$ $$=1\frac{3}{4}$$
Choice F
$$\frac{25^{x}}{5^{2}}=5^{4} $$ $$\Rightarrow \frac{5^{2x}}{5^{2}}=5^{4} $$ $$\Rightarrow 2x-2=4 $$ $$\Rightarrow 2x=6 $$ $$\Rightarrow x=3 $$
Choice B
$$f(x)=5\cdot 2^{x}$$ $$g(x)=10x$$ $$f(3)=5\cdot 2^{3}=5\cdot8=40$$ $$g(3)=10\cdot3=30$$ $$\Rightarrow f(3)-g(3)=40-30=10$$
Choice F
$$$5\ bill:x$$ $$$10\ bill:y$$ $$5x+10y=205---(1)$$ $$x+y=27---(2)$$ $$(1)-(2)\times5$$ $$\Rightarrow (5x+10y)-(5x+5y)=205-27\times5$$ $$\Rightarrow 5y=70$$ $$\Rightarrow y=14$$ $$\Rightarrow x=13$$
Choice H
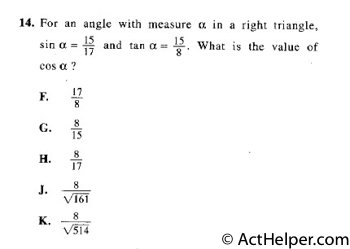
$$\sin^{2}(\theta)+\cos^{2}(\theta)=1$$ $$\Rightarrow \sin^{2}(\alpha)+\cos^{2}(\alpha)=1$$ $$\Rightarrow (\frac{15}{17})^{2}+\cos^{2}(\alpha)=1$$ $$\Rightarrow \frac{225}{289}+\cos^{2}(\alpha)=1$$ $$\Rightarrow \cos^{2}(\alpha)=\frac{64}{289}$$ $$\Rightarrow \cos(\alpha)=\sqrt{\frac{64}{289}}=\frac{8}{17}$$
Choice J
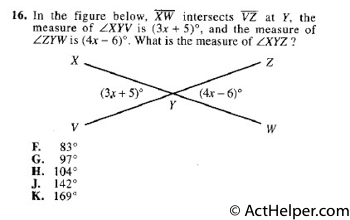
$$(3x+5)=(4x-6)$$ $$\Rightarrow x=5+6=11$$ $$\Rightarrow \angle XYV=3x+5=3 \times 11 +5=38°$$ $$\angle XYZ=180°-\angle XYV=180°-38°=142°$$
Choice E
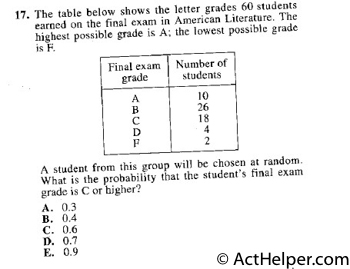
$$Grade\ C\ or\ higher:10+26+18=54$$ $$\Rightarrow\frac{Grade\ C\ or\ higher}{Total}=\frac{54}{60}=\frac{9}{10}=0.9$$
Choice F
$$x^{2}-2x+n=0$$ $$x=4,$$ $$\Rightarrow 4^{2}-2\cdot4+n=0$$ $$\Rightarrow 16-8+n=0$$ $$\Rightarrow n=-8$$ $$x=-2,$$ $$\Rightarrow (-2)^{2}-2(-2)+n=0$$ $$\Rightarrow 4+4+n=0$$ $$\Rightarrow n=-8$$
Choice E
$$Circumference = π × diameter = 2 × π × radius.$$ $$2\pi r=12 \pi $$ $$\Rightarrow r=6 $$ $$Area=\pi r^{2}=\pi 6^{2}=36\pi $$
Choice H
$$26-2=24$$ $$10-1=9$$ $$\Rightarrow24\times24\times24\times9\times9\times9$$
Choice E
$$3(a+b)-2(a-5b)$$ $$=3a+3b-2a+10b$$ $$=a+13b$$
Choice K

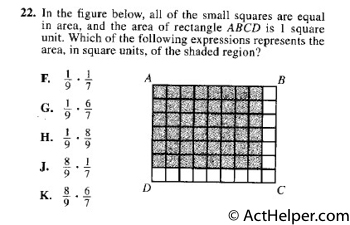
$$\Rightarrow\frac{8}{9}\times\frac{6}{7}$$
Choice D
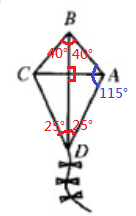
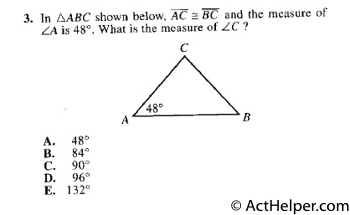
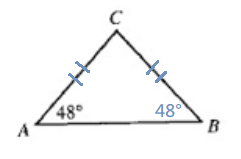
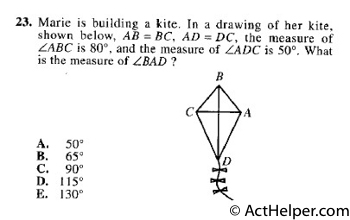
$$\overline{AB}=\overline{BC}$$ $$\angle CBD=\angle ABD=\frac{1}{2}\angle ABC=40°$$ …
Choice K
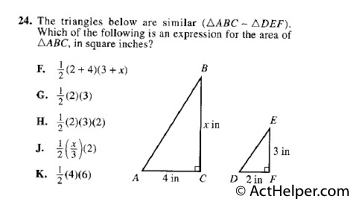
$$\because\triangle ABC\sim\triangle DEF$$ $$\therefore 2:4=3:x$$ …
Choice C
$$\because1\ foot =12\ inches$$ $$\therefore …
Choice F
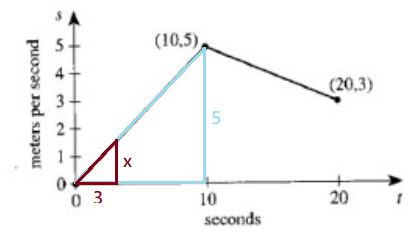
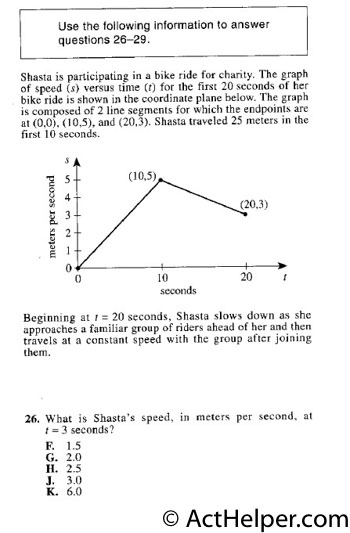
$$\Rightarrow 10:5=3:x$$ $$x=3\times\frac{1}{2}=1.5$$
Choice B
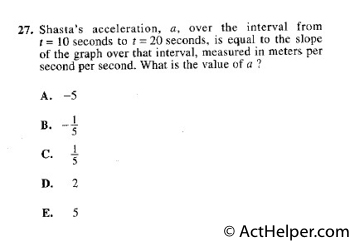
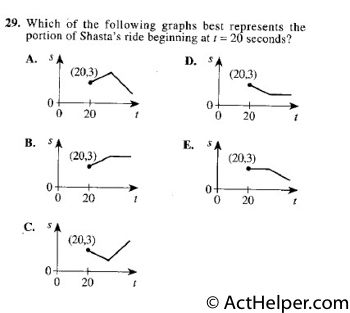
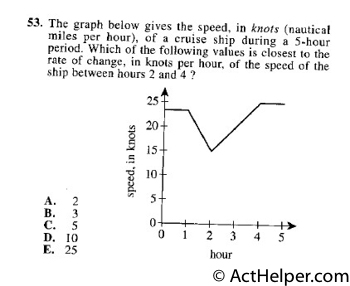
$$t=10,\ v=5$$ $$t=20,\ v=3$$ $$\frac{3-5}{20-10}=\frac{-2}{10}=-\frac{1}{5}$$
Choice H
$$Shasta's\ maximum\ speed:\ 5$$ $$\frac{1}{2}\times5\times15=37.5$$
Choice D

Choice J
$$\frac{2}{3}-\frac{5}{6}(\frac{2}{5}+\frac{1}{10})$$ $$=\frac{2}{3}-\frac{5}{6}(\frac{4}{10}+\frac{1}{10})$$ $$=\frac{2}{3}-\frac{5}{6}\times\frac{5}{10}$$ $$=\frac{2}{3}-\frac{5}{12}$$ $$=\frac{8}{12}-\frac{5}{12}$$ …
Choice E
$$(x+2)(2x+7)$$ $$=2x^{2}+7x+4x+14$$ $$=2x^{2}+11x+14$$
Choice H
$$F.\ 2\lt\sqrt{3}\lt4 $$ $$\Rightarrow 2=\sqrt{4} …
Choice E
$$P(Heads\ up)=\frac{1}{2}$$ $$\Rightarrow \frac{1}{2}\times\frac{1}{2}=\frac{1}{4}$$
Choice G
$$\frac{88\times6+2x}{8}=90$$ $$\Rightarrow 528+2x =720$$ $$\Rightarrow …
Choice C
$$A.\ (62+(-\frac{1}{65}))\div2=31-\frac{1}{130}$$ $$B.\ 62\div(-\frac{1}{65})=-62\times65=4030$$ $$\checkmark …
Choice F
$$A:\left\{0,1,2,3\right\}$$ $$B:\left\{1,3,5,7\right\}$$ $$F.\ f(x)=2x+1$$ $$f(0)=2\times0+1=1$$ …
Choice D
$$x=\frac{3}{4}+\frac{4}{3}=\frac{9+16}{12}=\frac{25}{12}$$ $$y=\frac{2}{3}+\frac{3}{2}=\frac{4+9}{6}=\frac{16}{6}=\frac{32}{12}$$ $$z=1+1=\frac{24}{12}$$ $$\Rightarrow \frac{32}{12}\gt\frac{25}{12}\gt\frac{24}{12}$$ …
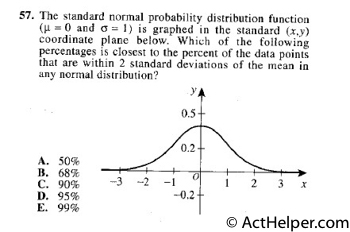
Choice G
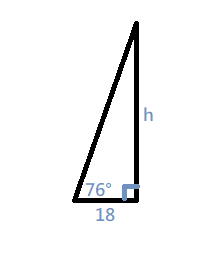
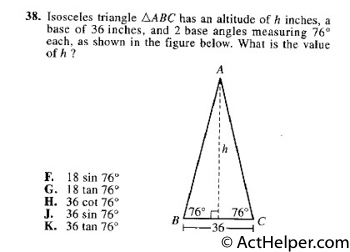
$$tan\ 76°=\frac{h}{18}$$ $$h=18\times tan\ 76°$$
Choice E
$$216=2^{3}\times 3^{3}$$ $$108=2^{2}\times 3^{3}$$ $$(A).[2,108]=108$$ …
Choice J
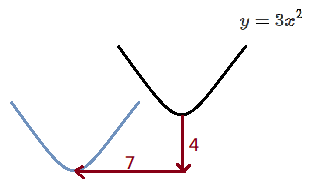
$$\Rightarrow y=3(x+7)^{2}-4$$
Choice E
$$Less\ than\ $100$$ $$\Rightarrow(Less\ than\ …
Choice G
$$At\ least\ $150\ each\ week$$ …
Choice C
$$$300\ or\ more:\ 0.1$$ $$\Rightarrow …
Choice F
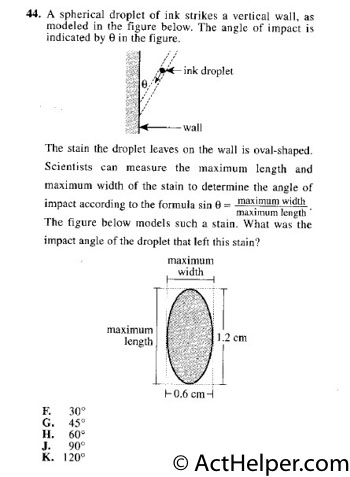
$$\sin\theta=\frac{maximum\ width}{maximum\ length}$$ $$=\frac{0.6}{1.2}=\frac{1}{2}$$
Take …
Choice A
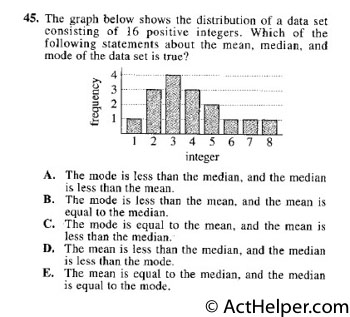
$$1,2,2,2,3,3,3,3,4,4,4,5,5,6,7,8$$ $$Mode=3$$ $$Mean=\frac{1+2\times3+3\times4+4\times3+5\times2+6+7+8}{16}$$ $$=\frac{1+6+12+12+10+6+7+8}{16}$$ $$=\frac{62}{16}=3.875$$ …
Choice K
$$Yolanda:\ y$$ $$Xavier:\ x$$ $$Zach:\ …
Choice D
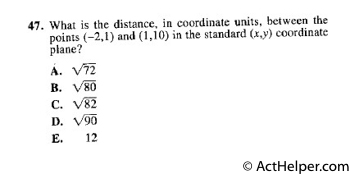
$$1-(-2)=3$$ $$10-1=9$$ $$\sqrt{3^{2}+9^{2}}=\sqrt{9+81}=\sqrt{90}$$
Choice J
$$100=10\times10\times1$$ $$\Rightarrow 20\times20\times2=800$$
Choice D
$$\mid x-2 \mid\lt7$$ $$\Rightarrow -7\lt …
Choice G
$$380,204,032$$ $$F.\ 1\ and\ 10$$ …
Choice B
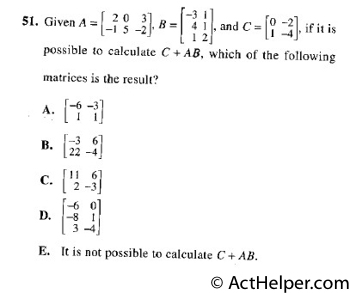
$$AB$$ $$=\begin{bmatrix}2(-3)+0(4)+3(1) & 2(1)+0(1)+3(2) \\(-1)(-3)+5(4)+(-2)1 …
Choice H
$$\frac{x+y}{y}$$ $$=\frac{x}{y}+\frac{y}{y}$$ $$=\frac{x}{y}+1$$
Clearly that …
Choice C
$$t=2\ hours,\ v_{2}=15$$ $$t=4\ hours,\ …
Choice K
$$(1).\ 1,2,3,4 \rightarrow Odd:1,3$$ $$(2).\ …
Choice A
$$Area=630\ square\ feet$$ $$Depth=5\ ft$$ …
Choice K
$$3!=3\times2\times1$$ $$N!= N(N-1)(N-2)...1$$ $$\Rightarrow (a!)^{b}=[a(a-1)(a-2)...1]^{b}$$
Choice D
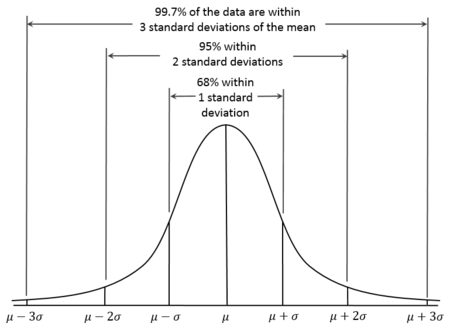
Choice G
$$\begin{bmatrix}a & b \\c & …
Choice C
$$x^{2}-2x+17=0$$ $$\frac{-b\pm\sqrt{b^{2}-4ac}}{2a} $$ $$\Rightarrow \frac{-(-2)\pm\sqrt{(-2)^{2}-4\times1\times17}}{2\times1} …
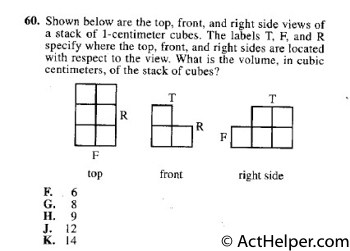
Choice G