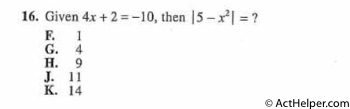Choice B
$$60=2\times2\times3\times5$$ $$84=2\times2\times3\times7$$ $$126=2\times3\times3\times7$$ $$(64,84,126)=2\times3=6$$
Choice C
$$$88\times\frac{3}{4}=$66$$ $$($20\times4)-($66\times1.07)$$ $$=$80-$70.62$$ $$=$9.38$$
Choice J
$$82\times$1+x\times$0.5=$140$$ $$\Rightarrow 0.5x=140-82=58$$ $$\Rightarrow x=116$$
Choice D
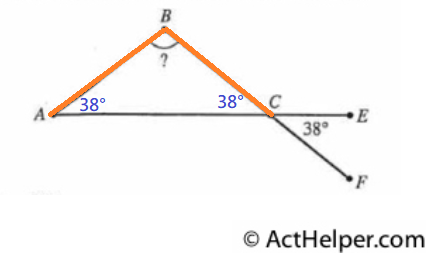
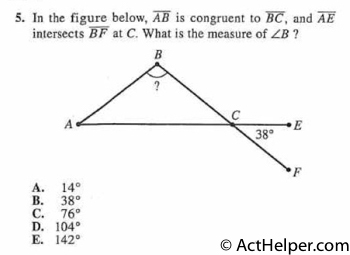
$$\because \overline{AB}=\overline{BC}$$ $$\therefore \angle BAC=\angle BCA=38°$$ $$\Rightarrow \angle B=180°-38°-38°=104°$$
Choice G
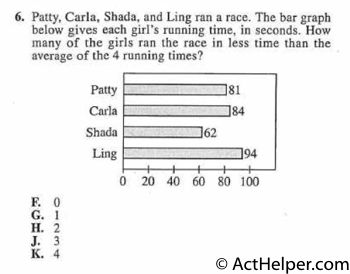
$$(81+84+62+94)\div4=80.25$$
Only one girl ran the race in less time than the average of the 4 running times.
Choice D
From 9:00 a.m. to 10:20 a.m., a total of 80 minutes.
$$\frac{18000}{80}=225$$Choice B
$$5-\frac{1}{2}-1\frac{3}{4}$$ $$=5-\frac{2}{4}-1\frac{3}{4}$$ $$=2\frac{3}{4}$$
Choice G
$$r=-3(4)+2$$ $$r=-12+2$$ $$r=-10$$
Choice A
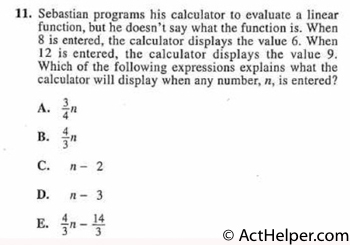
$$f(x)=mx+b$$ $$6=m(8)+b---(1)$$ $$9=m(12)+b---(2)$$ $$(2)-(1)$$ $$\Rightarrow 3=4m$$ $$\Rightarrow m=\frac{3}{4}$$ $$f(x)=\frac{3}{4}x+b$$ $$6=6+b$$ $$b=0$$ $$f(n)=\frac{3}{4}n$$
Choice C

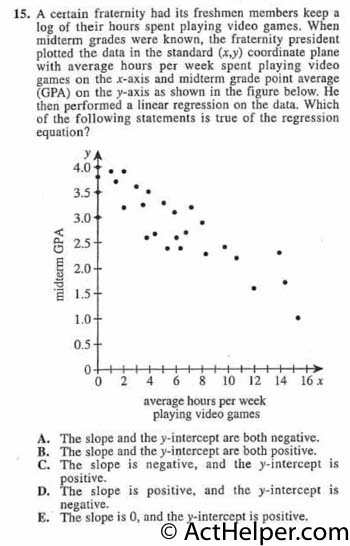
The slope is negative and intersects the Y axis at a position where Y is greater than 0, so the Y axis intercept is positive.
Choice G
$$4x+2=-10$$ $$\Rightarrow 4x=-12$$ $$\Rightarrow x=-3$$ …
Choice B
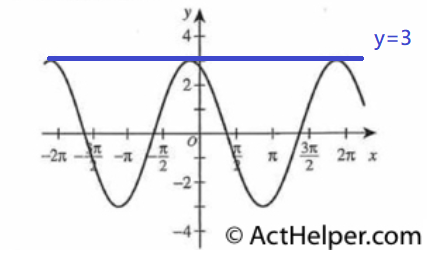
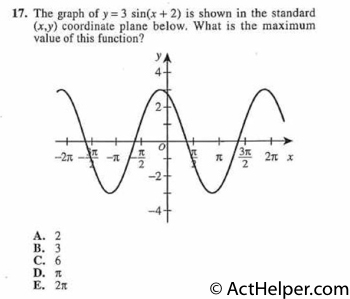
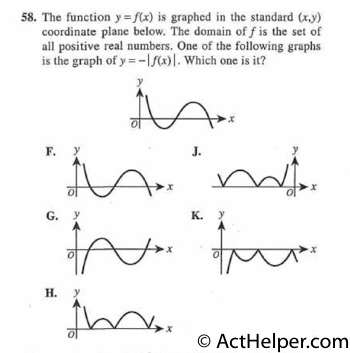
According to the picture provided, it can be observed that the maximum value of this function, that is, the maximum value of Y is 3.
Choice F
The mean is the average of the numbers.
The Median is the "middle" of a sorted list of numbers.
The mode is the number that is repeated more often than any other.
$$6,7,7,6,8,7,8,10,9$$Rearrange the 9 scores in order.
$$\rightarrow 6,6,7,7,7,8,8,9,10$$ $$Mean=(6+6+7+7+7+8+8+9+10)\div9=68\div9\approx7.55$$ $$Median=7$$ $$Mode=7$$After correcting the 9th score to 10.
$$\rightarrow 6,6,7,7,7,8,8,10,10$$ $$Mean=(68+1)\div9\approx7.66$$ $$Median=7$$ $$Mode=7$$Only the mean is changed.
Choice E
Parallel lines have the same slope and will never intersect.
$$y=\frac{2}{3}x+4$$ $$\Rightarrow Slope=\frac{2}{3}$$Only the E option has the same slope.
Choice E

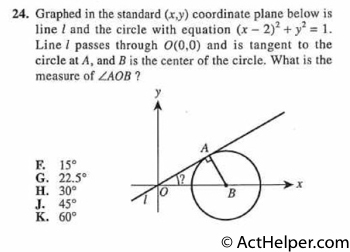
It's very clear after drawing …
Choice J
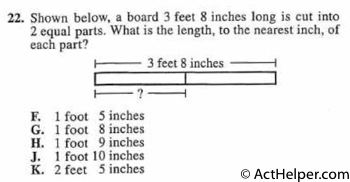
1 foot is equal to …
Choice E
1 foot is equal to …
Choice H

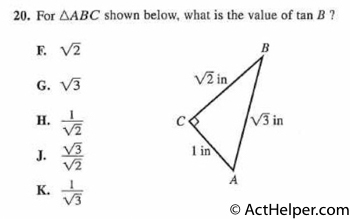
$$2^{2}=1^{2}+x^{2}$$ $$\Rightarrow x=\sqrt{3}$$ $$1:\sqrt{3}:2$$
It …
Choice E
$$x\times x+(x-2)(x-2)$$ $$=x^{2}+x^{2}-4x+4$$ $$=2x^{2}-4x+4$$
Choice H
$$\frac{5}{2}x+\frac{3}{4}y=-\frac{1}{2}$$ $$\Rightarrow 4\times\frac{5}{2}x+4\times\frac{3}{4}y=4\times(-\frac{1}{2})$$ $$\Rightarrow 10x+3y=-2$$ …
Choice D
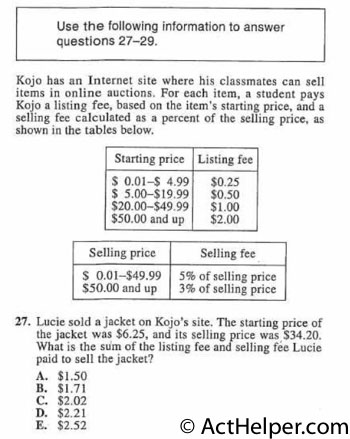
$$0.5+34.2(0.05)$$ $$=0.5+1.71$$ $$=2.21$$
Choice F
$$5.75\div0.5=11.5$$
Choice D
$$x+y=116---(1)$$ $$0.03x+0.05y=4.34---(2)$$ $$(1)\times5-(2)\times100$$ $$(5x+5y)-(3x+5y)=580-434$$ $$2x=146$$ …
Choice J
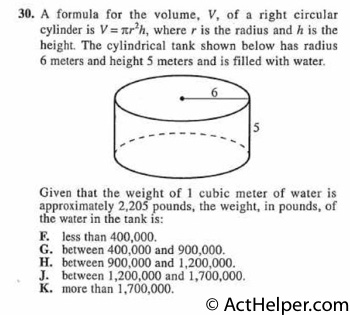
$$V=\pi r^{2}h$$ $$V=3.14(6)^{2}(5)=565.2$$ $$565.2\times2,205=1,246,266$$
It's …
Choice C
$$Children: x$$ $$Adults: y$$ $$x+y=21---(1)$$ …
Choice K
$$(\frac{2x^{3}y^{-5}z^{8}}{8x^{-2}y^{6}z^{3}})^{-2}$$ $$=(\frac{2}{8})(\frac{x^{3}y^{-5}z^{8}}{x^{-2}y^{6}z^{3}})^{-2}$$ $$=(\frac{1}{4})(x^{3-(-2)}y^{-5-6}z^{8-3})^{-2}$$ $$=(2^{-2})(x^{5}y^{-11}z^{5})^{-2}$$ $$=(2^{(-2)(-2)})(x^{5(-2)}y^{(-11)(-2)}z^{5(-2)})$$ …
Choice D
$$g(-1)=(-1)^{2}-1=1-1=0$$ $$f(g(-1))=f(0)=2(0)+3=3$$
Choice J
Choose a pot from the …
Choice B
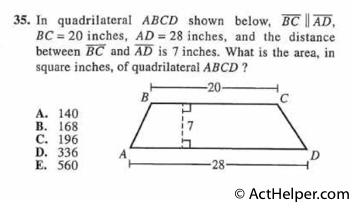
Area of a trapezoid is …
Choice H
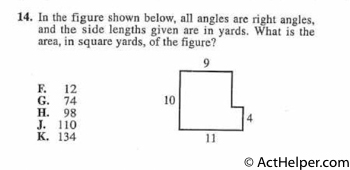
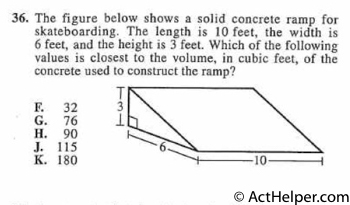
First calculate the area of …
Choice A
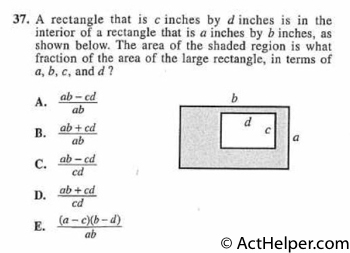
$$The\ large\ rectangle=ab$$ $$The\ shaded\ …
Choice F
$$Code\rightarrow A-H-Number-Number-Number-Number$$ $$Number:(1,2,3,4,5,6,7,8,9,0)$$ $$\Rightarrow 1(1)(10)(10)(10)(10)$$
Choice A
$$2\div5=0.4$$
Choice K
The box cotains 6 objects. …
Choice A
$$\log_{4}{x}=-3$$ $$\Rightarrow \log_{4}{4^{-3}}=-3$$ $$\Rightarrow x=4^{-3}=\frac{1}{64}$$
Choice J

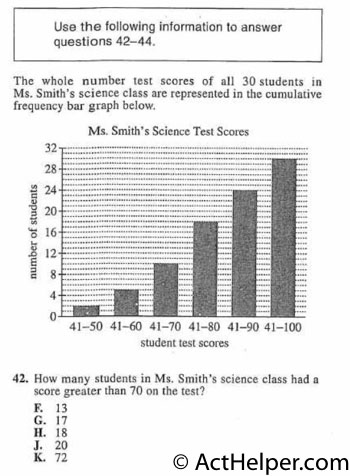
$$41-50\rightarrow 2$$ $$51-60\rightarrow 3$$ $$61-70\rightarrow …
Choice D

The Median is the "middle" …
Choice F
$$4\times2=8$$ $$8-3=5$$
Choice B
$$x-1=x-1$$ $$-(x-1)=x-1$$ $$-x+1=x-1$$ $$\Rightarrow x=1$$ …
Choice F
We require that the value …
Choice C

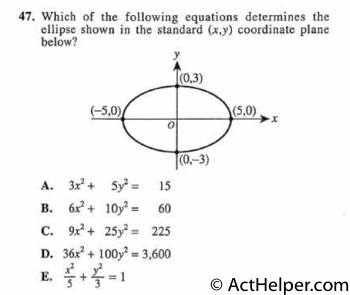
$$\frac{x^{2}}{a^{2}}+\frac{y^{2}}{b^{2}}=1$$ $$\Rightarrow \frac{x^{2}}{5^{2}}+\frac{y^{2}}{3^{2}}=1$$ $$\Rightarrow 9x^{2}+52y^{2}=1\times9\times25$$ …
Choice F
$$(-2)^{2}+(-2)b-6=0$$ $$\Rightarrow 4-2b-6=0$$ $$\Rightarrow -2=2b$$ …
Choice C

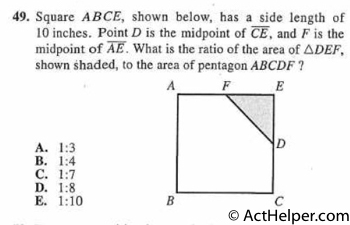
$$\Rightarrow 1:7$$
Choice G
$$12\div2=6$$ $$1+2+3=6$$ $$\mid-3\mid+\mid-2\mid+\mid-1\mid+\mid0\mid+\mid1\mid+\mid2\mid+ \mid3\mid$$ $$=3+2+1+0+1+2+3$$ …
Choice C

$$f(g(x))$$ $$=\sqrt{(x-3)^{3}}+1$$
If x < 3, you would be taking the square root of a negative number, so x ≥ 3.
Choice G
$$(0,0)$$ $$\rightarrow(0+4,0)=(4,0)$$ $$\rightarrow(4,0-1)=(4,-1)$$ $$\rightarrow(4+2,-1)=(6,-1)$$ $$\rightarrow(6,-1-6)=(6,-7)$$ …
Choice B
A Rational Number can be …
Choice K
Let X be -0.5 and …
Choice E

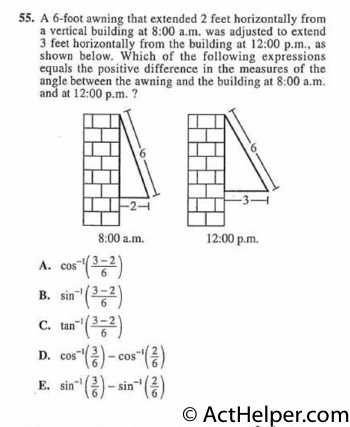
$$8:00\ a.m.\ \rightarrow \sin \theta=\frac{2}{6}$$ …
Choice K
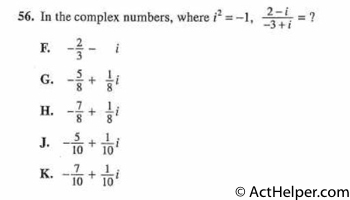
$$\frac{2-i}{-3+i}$$ $$=\frac{(2-i)(-3-i)}{(-3+i)(-3-i)}$$ $$=\frac{-6-2i+3i+i^{2}}{(-3)^{2}-(i)^{2}}$$ $$=\frac{-6+i-1}{9-(-1)}$$ $$=\frac{(-7+i)}{10}$$ …
Choice E
The sum of the internal …
Choice K

The absolute value sign is …
Choice D
$$The\ clearance\ price$$ $$=The\ sale\ …
Choice F
$$2\pi r=2 \pi\times2=4\pi$$ $$\frac{4\pi}{4}=\pi$$