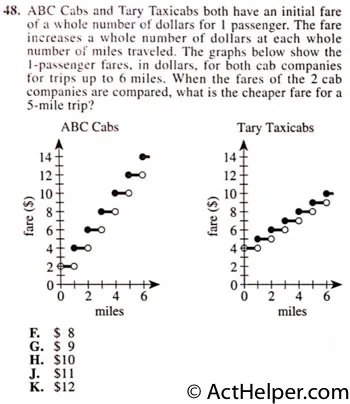
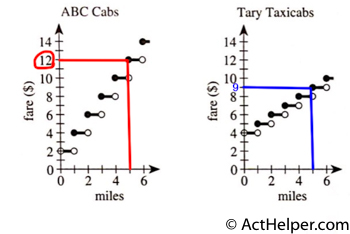
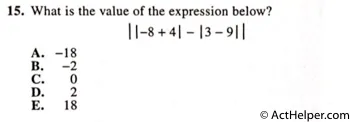Choice B
$$x=\frac{12}{8}=\frac{3}{2} \\$$ $$ y=\frac{22-10}{2}=6 \\ $$ $$\Rightarrow x +y=7\frac{1}{2}$$
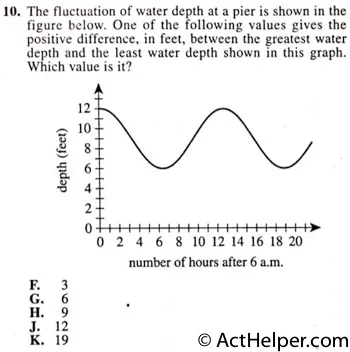
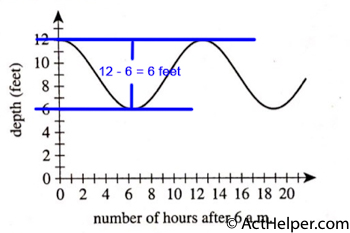
Choice H
Median = Average = 420 ÷ 5 = 84
→ sum of the 4 scores that are NOT the median = sum - median = 420 - 84 = 336
Choice B
By rearranging the equation into slope intercept form, we have: y =(4/7)x-(5/7) Therefore the slope is 4/7.Choice K
The sum of an odd integer and an even integer will always be an odd integer. For example, 1 + 2 = 3
Choice K
It is not given that the triangle is a right triangle, so you can’t use the Pythagorean theorem. The length of DF ranges from, not inclusively, (√30-3) to (√30+3).
Choice J
$$14x\geq 175 \\$$ $$ \Rightarrow x \geq 12.5 \\ $$ $$\Rightarrow x_{min}=13$$
Choice A
$$\frac{4.8\times10^{-7}}{1.6\times10^{-11}}=3.0\times 10^{-7+11}=3.0\times 10^{4}$$
Choice H
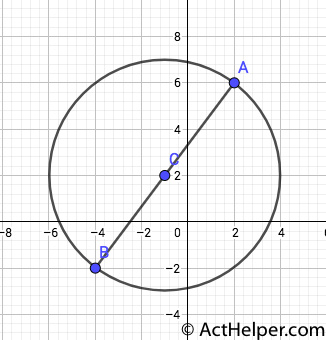
Vector CA which points from C to A has components given as <3, 4>. Thus, the vector that points from C to B = -CA = <-3, -4>. Hence, the coordinates of point B is (-4,-2).
Choice J
There is 7/9 of the original pizza remaining. Thus, each brother will get (1/3)(7/9) = 7/27 of the original pizza.
Choice E
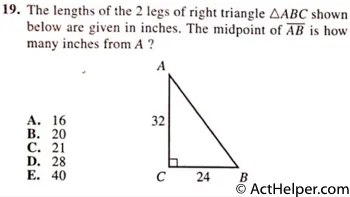
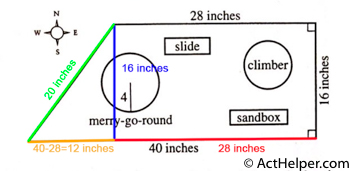
$$Length \ of green \ side=\sqrt{16^2+12^2}=20 \\$$ $$ \Rightarrow Perimeter = 20+28+16+40=104 \ inches = 104\times 1.5 \ feet = 156 \ feet$$
Choice C
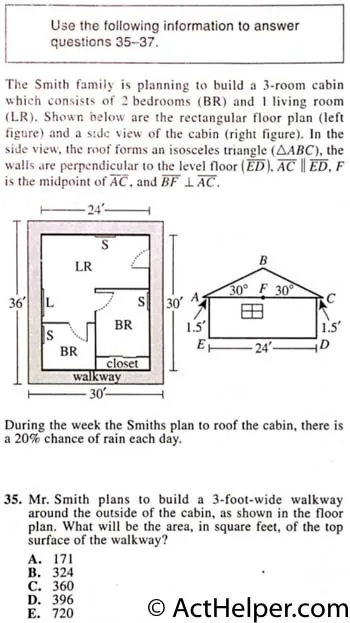
The area of the walkway can be arrived at by deducting the area of bigger rectangular (30’ x 36’) from that of the smaller rectangular (30’ x 24’):
Area of walkway = 30’ x 36’ - 30’ x 24’ = 360 square feet
Choice J
There are 3 rooms so 3 fans will cost 3($52) = $156. There are 3 small and 1 large windows so the total cost of the curtains will be 3($39.50)+1($79) = $197.50. Therefore, the total cost is $156+$197.50 = $353.50.
Choice A
Because the chance of raining on a particular day is independent of the chance of rain on another day, the probability that it rains two consecutive days is (0.2)(0.2) =0.04
Choice K
An irrational number cannot be written as a ratio of two integers. (F) evaluates to √(1/4) = 1/2. (G) evaluates to √(4) = 2 = 2/1. (H) evaluates to 2 = 2/1. (J) evaluates to √(16) = 4 = 4/1. (K) cannot be simplified further and cannot be written as a ratio of two integers.
Choice K
$$|2x-8|=2 \\$$ $$ \Rightarrow 2x-8=2 \ or \ 2x-8=-2 \\$$ $$ \Rightarrow x=5 \ or\ x=3$$
Choice A
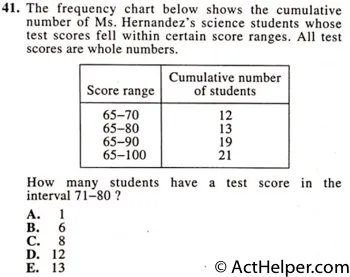
There are 12 students who have scores between 65-70 but 13 who have scores in that range plus the range of 71-80 (totalling a range of 65-80). Thus, there are 13-12 = 1 student with scores between 71-80.
Choice C
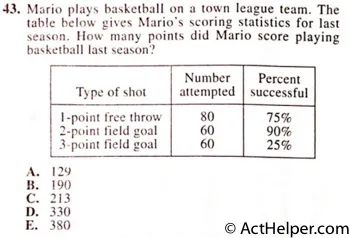
$$score = 1\times 80 \times 75 \% + 2 \times60 \times90 \%+3\times60 \times 25\%=213$$
Choice F
The graph is moved to the right for n units whenever x is substitute for x - n.
Choice A
The volume of the toy soldier can be found by deducting the volume of the rectangular container after the submerging of the toy soldier from that before:
Volume of toy soldier = 8 x 6 x 6.6 – 8 x 6 x 4 = 125
Choice J
Volume of the box = 18³
Volume of the cube = π(6)(12)²
--> Volume of packing material = 18³ - π(6)(12)²
Choice B
1 yard = 3 feet
1 feet = 12 inches
Area of the floor = 15 feet x 21 feet = 5 yard x 7 yard = 35 square yards
Choice B
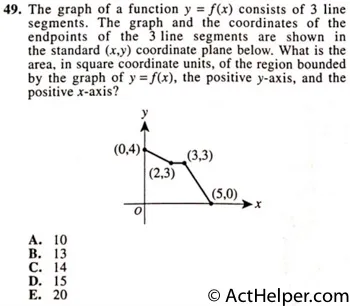
The area is simply the area of the trapezoid spanned from x = 0 to 2 plus the area of the rectangle from x = 2 to 3 plus the area of the triangle from x = 3 to 5. Thus, the area = .5(4+3)(2)+(1)(3)+.5(3)(2) = 13
Choice J
x + y = 151
x=19+√y
--> x²-37x+210=0
--> (x-7)(x-30)=0
--> x = 7 or 30
If x = 7, √y<0, therefore x cannot be 7
--> x = 30, y = 121
-->y-x = 91
Choice C
The list of number is ordered from the highest to the lowest.
The median of the list is 25, therefore (30+X) / 2 = 25 –> X = 20
The mode of the list is 15, therefore Y = 15
The mean of the list = (41+35+30+20+15+15) / 6 = 26
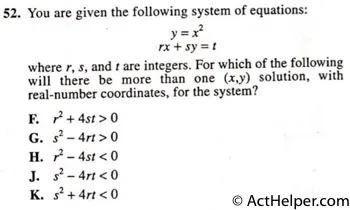
Choice F
Substituting y for x², we have
s·x² + r·x – t = 0
For the equation to have two different solutions,
Δ = r² + 4st › 0
Choice A
$$a_{3}=13 \\ a_{4}=18 \\$$ $$ \Rightarrow d=a_{4}-a_{3}=5 \\$$ $$ \Rightarrow a_{50}= a_{4} +(50-4)\times d =248$$
Choice H
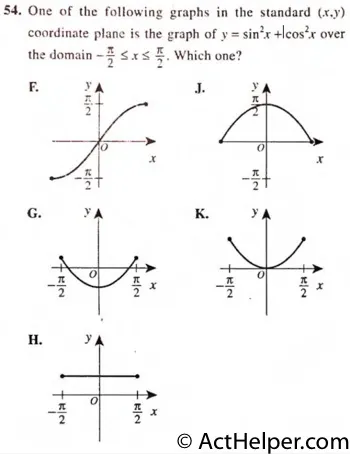
The Pythagorean trigonometric identity is expressed as:
sin² x + cos² x = 1
Therefore, y = sin² x + cos² x = 1
Choice E
The regular period of csc(x) is 2π. Thus, csc(4x) represents a compression of csc(x) towards the y axis by a factor of 4. Thus, the period will be 2π/4 = π/2
Choice H
For the toss of a penny, the probability of it landing with its head faceup is 50%. If it lands with its head up, the awarded value is 3 points. Otherwise the point is 0. Therefore the expected value of the point awarded for the toss of a penny is 3 x 50% + 0 x 50% = 3/2.
The same can also be said of the toss of a nickel and a dime. Therefore the expected value of the total points awarded is 3/2 +3/2 +3/2 = 9/2.
Choice B
We wish to find k for which k2-12 = k. Rearranging yields k2-k-12 = (k-4)(k+3) = 0. Thus, k = 4
Choice F
If i² = -1, then i^4 = (-1)² = 1. And notice that (i^4)k = i^4k = 1k = 1, k ∈ ℤ. In other words, i^4 = i^8 = i^12 = … = 1. Thus, we may conclude that 4|n (4 divides n; you do not need to know this notation).
Choice A
Recall that |sinθ| ≤ 1 for all θ ∈ R. Thus, we need only to find θ where sinθ = ±1. Going back to your unit circle, those values are θ = ±π/2
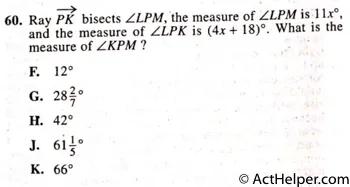
Choice K
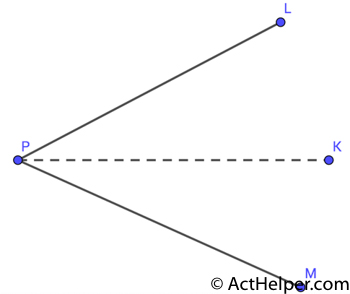
∠LPK · 2 =∠LPM
->(4x + 18°) · 2 = 11x
-> x = 12°
-> ∠KPM = 4· 12° + 18°=66°