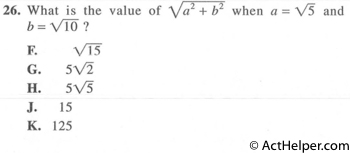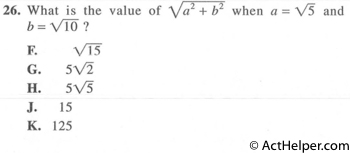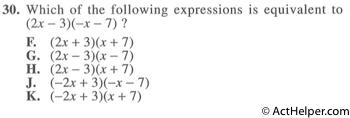Choice A
it says that "Danica will donate $35.00, plus $0.07 for each book donated by her classmates.", and that her classmates donated b books:
d = 35 + 0.07b
Choice D
| Rule name | Rule | Example |
|---|---|---|
| Product rules | a n ⋅ a m = a n+m | 23 ⋅ 24 = 23+4 = 128 |
| a n ⋅ b n = (a ⋅ b) n | 32 ⋅ 42 = (3⋅4)2 = 144 | |
| Quotient rules | a n / a m = a n–m | 25 / 23 = 25-3 = 4 |
| a n / b n = (a / b) n | 43 / 23 = (4/2)3 = 8 | |
| Power rules | (bn)m = bn⋅m | (23)2 = 23⋅2 = 64 |
| bnm = b(nm) | 232 = 2(32)= 512 | |
| m√(bn) = b n/m | 2√(26) = 26/2 = 8 | |
| b1/n = n√b | 81/3 = 3√8 = 2 | |
| Negative exponents | b-n = 1 / bn | 2-3 = 1/23 = 0.125 |
| Zero rules | b0 = 1 | 50 = 1 |
| 0n = 0 , for n>0 | 05 = 0 | |
| One rules | b1 = b | 51 = 5 |
| 1n = 1 | 15 = 1 | |
| Minus one rule | (-1)n = -1 , n odd v (-1)n = 1 , n even |
(-1)5 = -1 |
| Derivative rule | (xn)‘ = n⋅x n-1 | (x3)‘ = 3⋅x3-1 |
| Integral rule | ∫ xndx = xn+1/(n+1)+C | ∫ x2dx = x2+1/(2+1)+C |
4b8⋅5b3 = 4⋅5⋅b8+3=20b11
Choice K
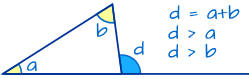
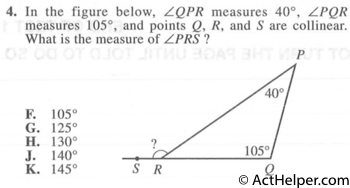
The Exterior Angle Theorem states that, for a triangle:
- The exterior angle d equals the angles a plus b
- The exterior angle d is greater than angle a, or angle b.
Therefore, ∠PRS = 40° + 105° = 145°
Choice D
Let the larger number be L, the smaller number be S:
L + S = 90
L = S + 50
⇒ S = 20, L = 70
Choice G
The absolute value of a number is its distance from zero on a number line.
|7 - 5| - |1 - 8| = 2 - 7 = -5
Choice G
$$(x^{2}+2x)+(3x+5)+(x^{2}+1)+(6x-4)$$ $$=(x^{2}+x^{2})+(2x+3x+6x)+(5+1-4)$$ $$=2x^{2}+11x+2$$
Choice E
Let the number of Tough Cuts be T, the number of Easy Pushes be E,
T + E = 96
T = 2E
⇒ E = 32, T = 64
Choice H
The median of a set of numbers is the middle number in the set (after the numbers have been arranged from least to greatest) -- or, if there are an even number of data, the median is the average of the middle two numbers.
In descending order, the set of numbers is:
116, 142, 175, 186, 191, 201, 201
Median = 186
Choice G
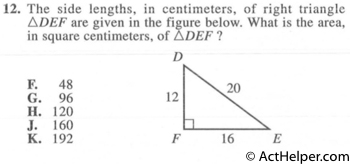
The area of a triangle is given by the equation:
Area△ = (1/2) × base × height
Therefore,
Area△ = (1/2) × 16 × 12 = 96
Choice H
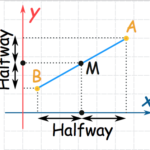
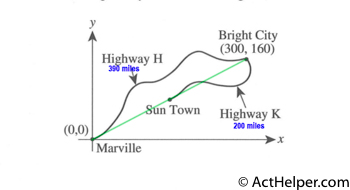
Midpoint of a Line Segment
The midpoint is halfway between the two end points:
- Its x value is halfway between the two x values
- Its y value is halfway between the two y values
To calculate it:
- Add both “x” coordinates, divide by 2
- Add both “y” coordinates, divide by 2
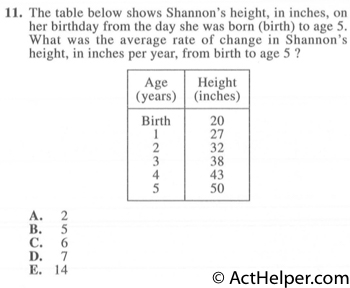
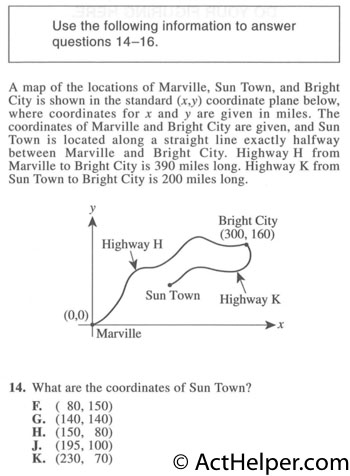
x = (1/2) × (0 + 300) = 150
y = (1/2) × (0 + 160) = 80
Choice C
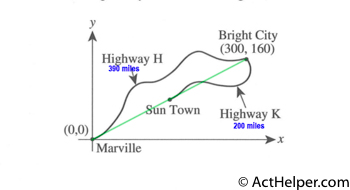
Distance between two points P(x1, y1) and Q(x2, y2) is given by:
$$d(P,Q) = \sqrt{(x_{2}-x_{1})^2+(y_{2}-y_{1})^2}$$Distance of a point P(x, y) from the origin is given by:
$$d(0,P) = \sqrt{x^2+y^2}$$Therefore,
$$d(0,Bright\ City) = \sqrt{300^2+160^2}= 340$$which is between 300 and 390.
Choice A
For any fraction, the greater its numerator and the lessor its denominator, the greater the value of the fraction.
Among the choices, d / a has the greatest numerator d and the least denominator a.
Choice J
Let the number of extra hours Maurice worked beyond 40 hours be E:
$12.5 × 40 + $18.75 × E = $931.25
⇒ E = 23
⇒ Total hours = 40 + 23 = 63 hours
Choice H
The perimeter of a parallelogram can be found by the formula:
perimeter = 2(w+h)
where:
w is the base length of the parallelogram
h is the side length
80 = 2 (18 + h)
⇒ h = 22
The other sides are: 18, 22, 22.
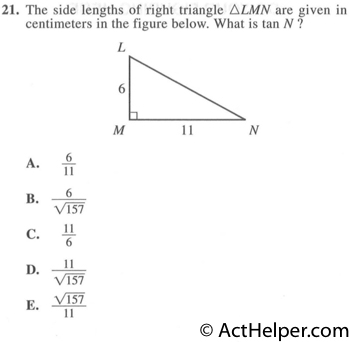
Choice A
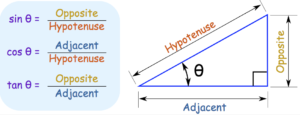
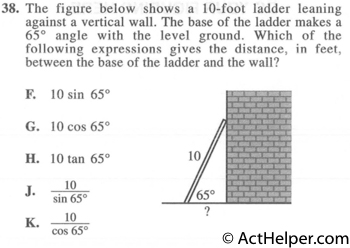
Thus, tan N = 6/11
Choice J
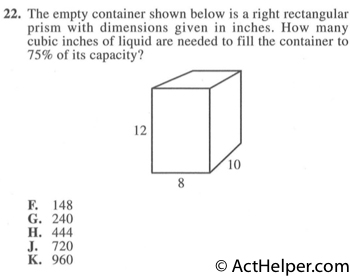
The volume of a right …
Choice A
The perimeter of a rectangle …
Choice F
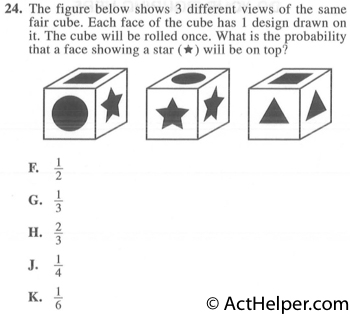
The cube has 2 stars …
Choice C
First off, we need to …
Choice F
Plugging the numbers into the …
Choice F
Plugging the numbers into the …
Choice B
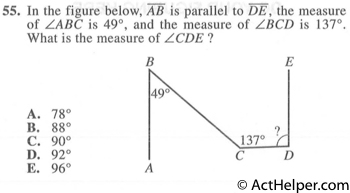
Two triangles are said to …
Choice K
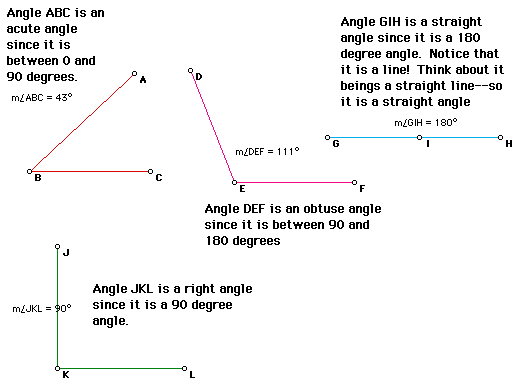
The measure of a straight …
Choice A
Given any two points on …
Choice K
(2x – 3)(-x – 7) …
Choice C
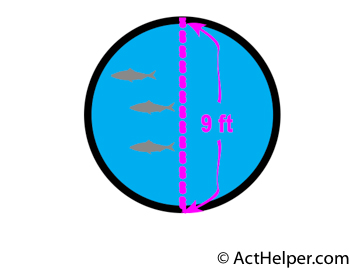
The perimeter of a circle …
Choice F

Since there are a total …
Choice E
The expression ” the product …
Choice J
The quadratic formula for the …
Choice C
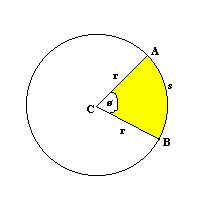
Generally, The area of a …
Choice F
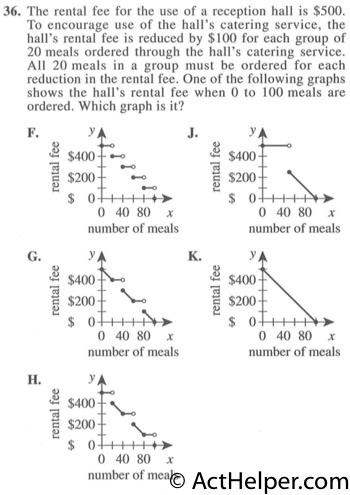
It says that “rental fee …
Choice A
Any line parallel to the …
Choice G
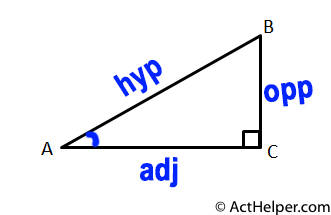
Let’s refresh the definitions of …
Choice C
Such integers can be:
1, …
Choice G
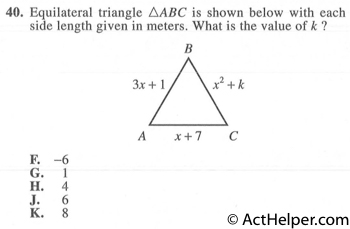
By definition, each side of …
Choice D
Simplifying the equation, we have: …
Choice F
$1.5 / 125 = $0.012 …
Choice C
Let’s divide all the possible …
Choice F
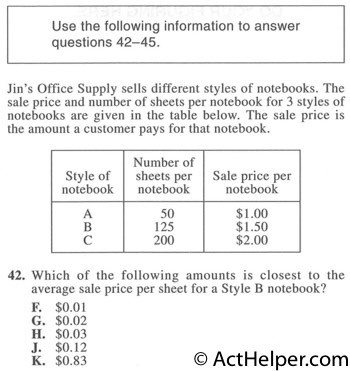
Let the number of Style …
Choice D
200 × 1.6 = 320
Choice J
3x + 5y = 17 …
Choice E
The least common multiple is …
Choice G
The slopes of two lines …
Choice A
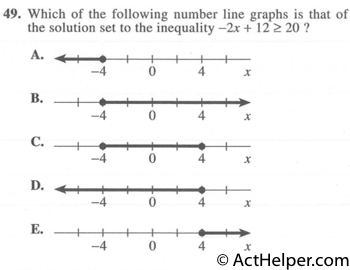
Graphing an inequality on a …
Choice G
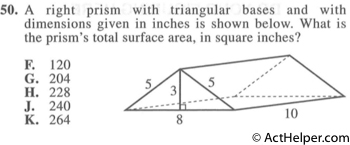
Total surface area = ½ …
Choice C
1 # 2 = 1 …
Choice G
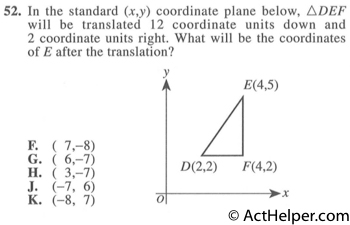
When a point is translated …
Choice C
What you need to know …
Choice K
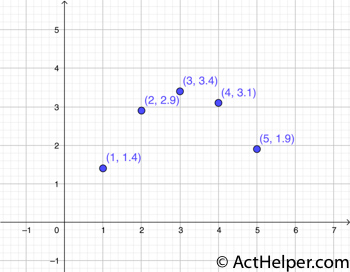
What is looks like is …
Choice D
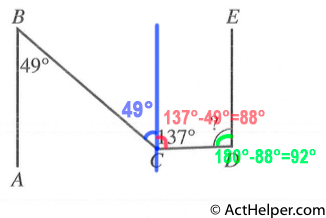
When parallel lines get crossed …
Choice G
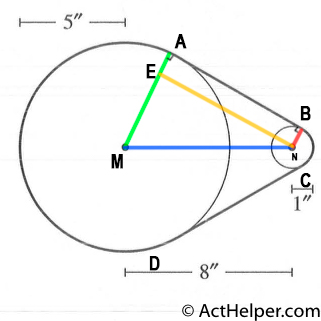
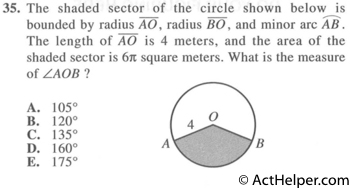
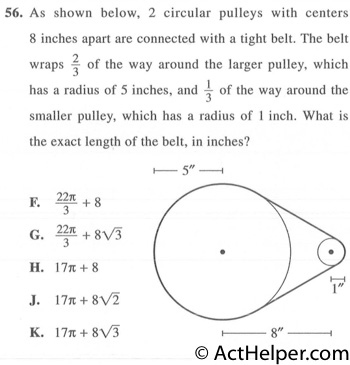
ME = AM – AE = AM – BN = 5 – 1 = 4;
MN = 8
⇒ AB = CD = EN = √(ME² + MN²) = 4√3
arc AMD = 2/3 ⋅ 2π ⋅ 5 = 20/3 ⋅ π
arc BNC = 1/3 ⋅ 2π ⋅ 1 = 2/3 ⋅ π
⇒ Length of the belt = arc AMD + AB + arc BNC + CD = 20/3 ⋅ π + 4√3 + 2/3 ⋅ π + 4√3 = 22/3 ⋅ π + 8√3
Choice E
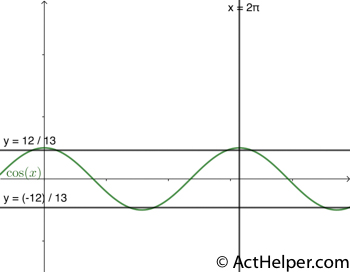
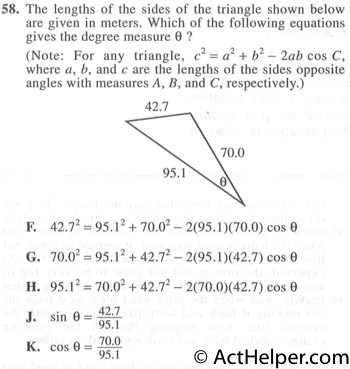
It should be remembered that:
sin2 A + cos2 A = 1
⇒ cos A = ± 12/13
As can be seen from the diagram, in the domain from 0 to 2π (0°-360°), it is possible for cos A to be negative.
Choice F
We should recognize that the …
Choice D
An arithmetic sequence is a …
Choice K
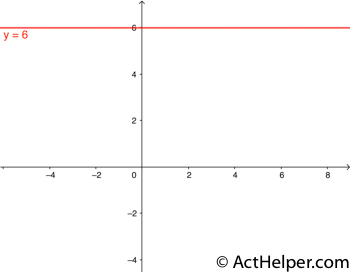
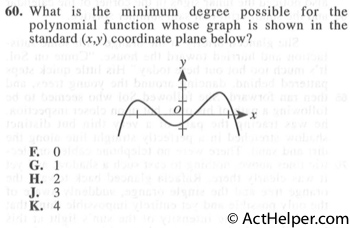
The graph crosses the x-axis …