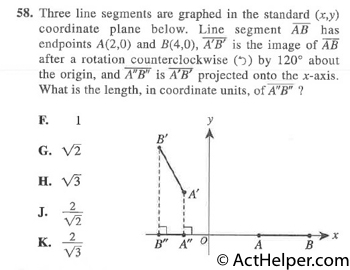
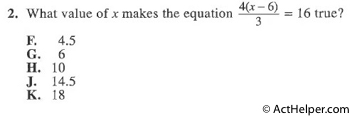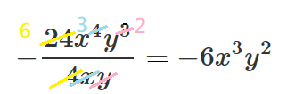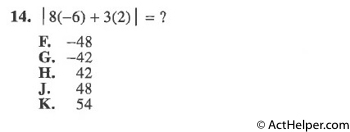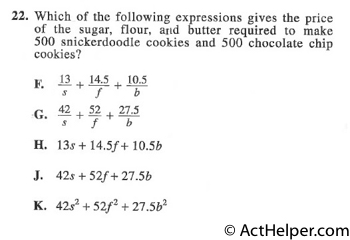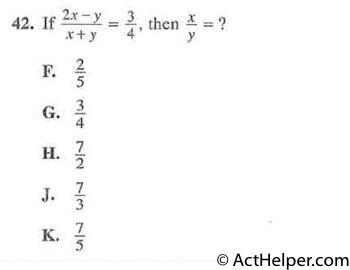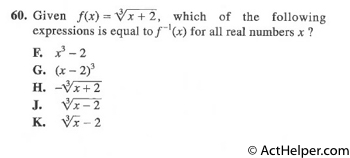Choice K
$$\frac{4(x-6)}{3}=16$$ $$\Rightarrow 4(x-6)=16\times3=48$$ $$\Rightarrow 4x-24=48$$ $$\Rightarrow 4x=72$$ $$\Rightarrow x=18$$
Choice J
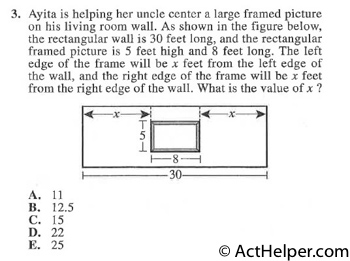
$$\Rightarrow 6x-3=3x+1$$ $$\Rightarrow 3x=4$$ $$\Rightarrow x=\frac{4}{3}$$
Choice G
$$=\frac{3\times4+1\times5}{3\times1-4\times5}$$ $$=\frac{12+5}{3-20}$$ $$=\frac{17}{-17}$$ $$=-1$$
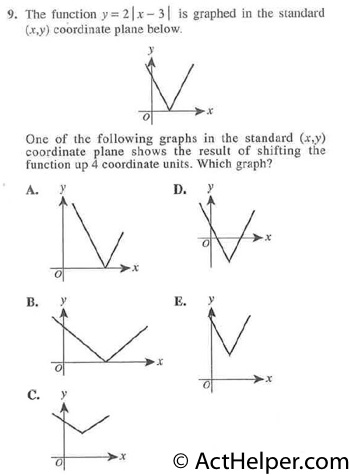
Choice E
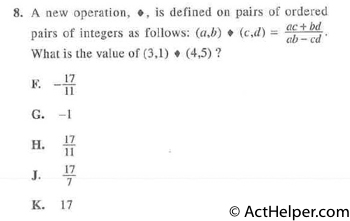
$$x=3,y=0\Rightarrow (3,0)$$ $$x=0,y=6\Rightarrow (0,6)$$ $$(3,0+4)=(3,4)$$ $$(0,6+4)=(0,10)$$
Choice G
$$21=3\times7$$ $$6=2\times3$$ $$4=2\times2$$ $$\Rightarrow 2\times2\times3\times7=84$$
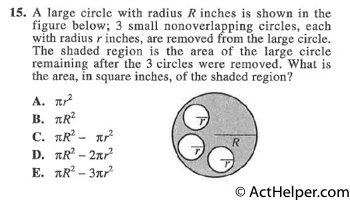
Choice E
$$The\ area\ of\ a\ circle=\pi r^{2}$$ $$\Rightarrow \pi R^{2}-3\pi r^{2}$$
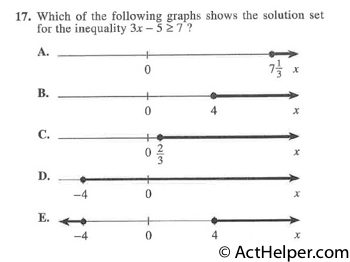
Choice B
$$3x-5\geq 7$$ $$\Rightarrow 3x\geq7+5$$ $$\Rightarrow x\geq \frac{7+5}{3}$$ $$\Rightarrow x\geq \frac{12}{3}$$ $$\Rightarrow x\geq 4$$
Choice C
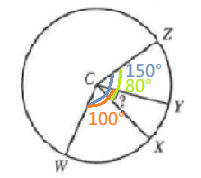
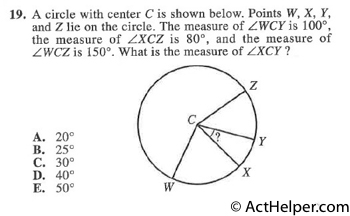
$$\angle WCZ-\angle WCY$$ $$150°-100°=50°=\angle YCZ$$ $$\angle XCY=\angle XCZ-\angle YCZ$$ $$ =80°-50°=30°$$
Choice A
$$\frac{27}{5}=5.4$$ $$\frac{24}{6}=4$$ $$\frac{20}{4}=5$$ $$4\times500=2000$$
Choice H
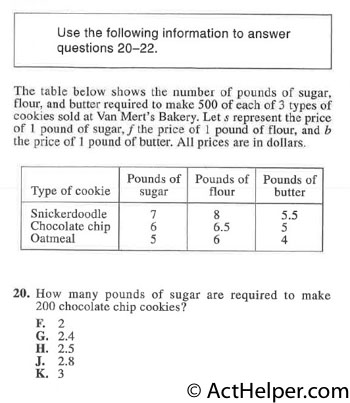
$$(7+6)s+(8+6.5)f+(5.5+5)b$$ $$=13s+14.5f+10.5b$$
Choice B
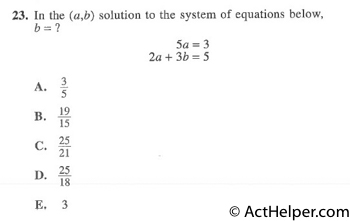
$$5a=3---(1)$$ $$2a+3b=5---(2)$$ $$(2)\times5-(1)\times2$$ $$\Rightarrow (10a+15b)-(10a)=5\times5-3\times2$$ …
Choice H
$$24\times150=3600$$ $$4\times60=240$$ $$3600\div240=15$$
Choice E
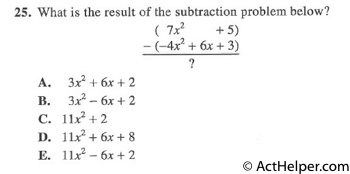
$$7-(-4)x^{2}-6x+(5-3)$$ $$=11x^{2}-6x+2$$
Choice J
$$(x^{2})^{3}(x^{4})^{5}=x^{a}$$ $$=x^{2\times3+4\times5}=x^{a}$$ $$6+20=a$$ $$a=26$$
Choice D
$$\frac{0.003}{100}=0.0003$$
Choice H
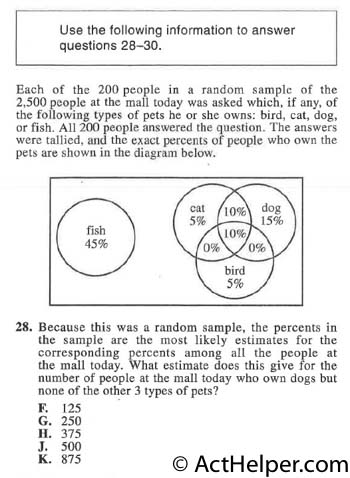
$$2500\times0.15=375$$
Choice D
$$45+5+15+5=70$$
Choice F
$$200\times0.45=90$$ $$\Rightarrow \frac{90}{200}=0.45$$ $$\Rightarrow \frac{90+15}{200+25}=\frac{105}{225}$$
Choice C
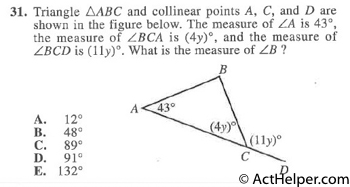
$$4y+11y=15y$$ $$y=\frac{180}{15}=12$$ $$4y=48$$ $$\angle B=180°-43°-48°=89°$$
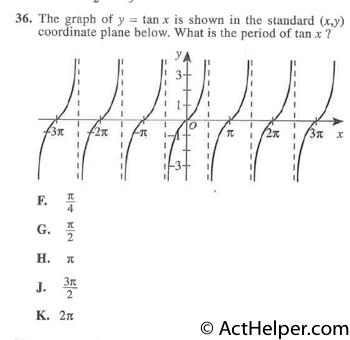
Choice F
$$x-\sqrt{5}=0$$ $$x=\sqrt{5}$$ $$y-1=0$$ $$y=1$$
Choice E
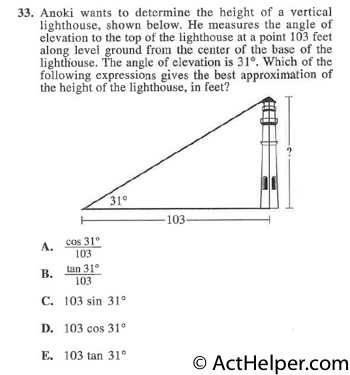
$$\tan 31°=\frac{?}{103}$$ $$?=\tan 31°103$$
Choice J
y=4 is a line parallel …
Choice B
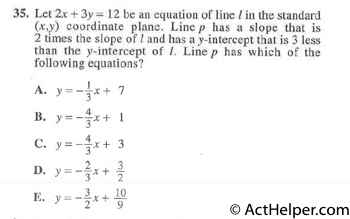
$$2x+3y=12---(line\ l)$$ $$\Rightarrow y=-\frac{2}{3}x+4$$ $$y=(-\frac{2}{3}\times2)x+(4-3)$$ …
Choice H
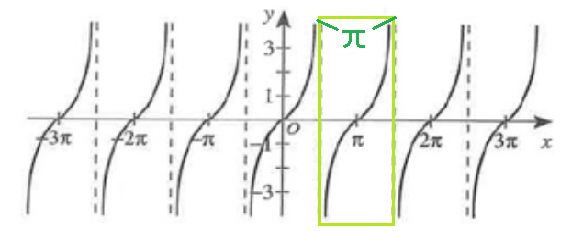
Choice C
$$5r\times2r=90$$ $$10r^{2}=90$$ $$r^{2}=9$$ $$r=3$$ $$3\times5=15$$
Choice G
As time elapses, the distance …
Choice B
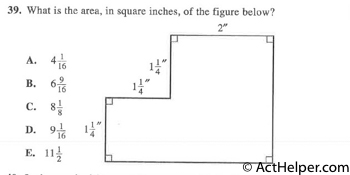
$$2\times2\times(1\frac{1}{4})+(1\frac{1}{4})(1\frac{1}{4})$$ $$5+\frac{25}{16}$$ $$=6+\frac{9}{16}$$
Choice K
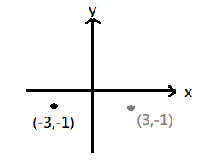
Choice D
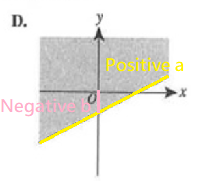
Choice K
$$\frac{2x-y}{x+y}=\frac{3}{4}$$ $$4(2x-y)=3(x+y)$$ $$8x-4y=3x+3y$$ $$5x=7y$$ $$\frac{x}{y}=\frac{7}{5}$$
Choice C
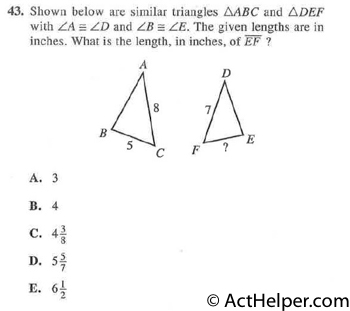
$$8:7=5:? $$ $$\frac{8}{7}=\frac{5}{?}$$ $$8\times?=5\times7$$ $$?=\frac{35}{8}=4\frac{3}{8}$$
Choice H
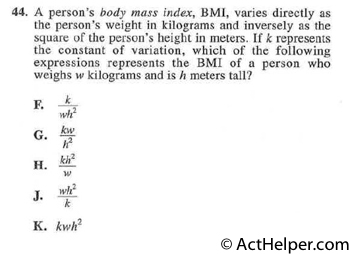
The formula is BMI = …
Choice E
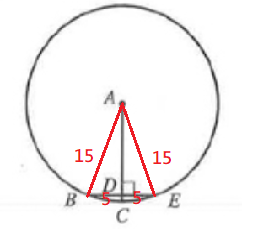
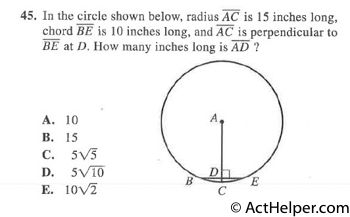
$$15^{2}-5^{2}=\overline{AD}^{2}$$ $$225-25=200=\overline{AD}^{2}$$ $$\overline{AD}=\sqrt{200}=10\sqrt{2}$$
Choice G
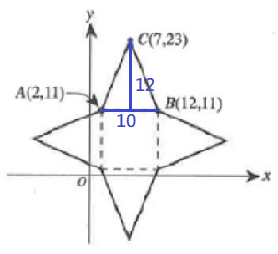
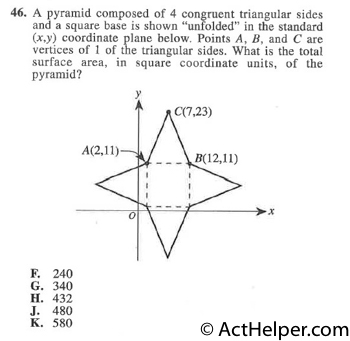
$$\triangle\times4+\Box$$ $$\Rightarrow\triangle=10\times12\times\frac{1}{2}=60$$ $$\Rightarrow\Box =10\times10=100$$ $$\triangle\times4+\Box=60\times4+100=340$$
Choice D
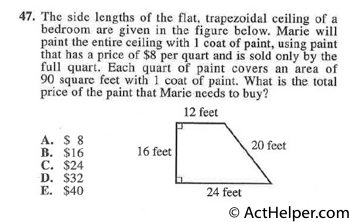
$$(12+24)\times16\times\frac{1}{2}$$ $$=36\times16\times\frac{1}{2}$$ $$=288$$ $$\frac{288}{90}=3...18$$
Marie …
Choice F
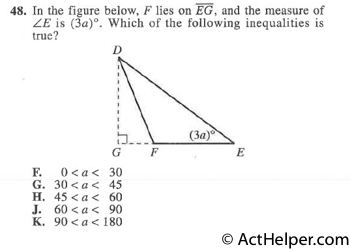
$$3a°\lt90°$$ $$a\lt30$$
Choice C
$$Correct\rightarrow 2x$$ $$Incorrect\rightarrow x$$ $$30+10(2x)-5(x)=150$$ …
Choice F
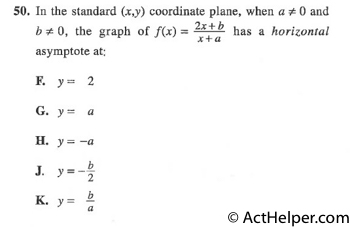
$$f(x)=\frac{2x+b}{x+a}$$ $$=\frac{2x}{x+a}+\frac{b}{x+a}$$ $$=\frac{2}{1+\frac{a}{x}}+\frac{\frac{b}{x}}{1+\frac{a}{x}}$$ $$\lim_{\frac{a}{x}\rightarrow0}\frac{2}{1+\frac{a}{x}}=2$$ $$\lim_{\frac{b}{x}\rightarrow0}\frac{\frac{b}{x}}{1+\frac{a}{x}}=0$$
Choice B
$$-0.43\lt-0.423\lt-0.42$$ $$\Rightarrow -\frac{43}{100}\lt-0.423\lt-\frac{42}{100}$$ $$n=-43$$
Choice F
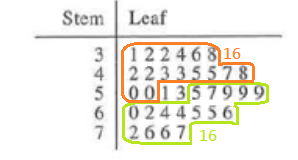
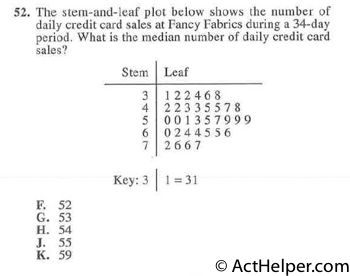
$$\frac{51+53}{2}=52$$
Choice D
$$\frac{25}{3}\pi=\frac{25\times180°}{3}=1500°$$ $$1500°=360°\times4+60°$$
Choice J
$$(6-7i)(\pi+6i)$$ $$=6\pi+36i-7i\pi+42$$ $$=(J)$$
Choice A
$$x=4,$$ $$4^{2}-4a-12=0$$ $$\Rightarrow 16-4a-12=0$$ $$\Rightarrow …
Choice J
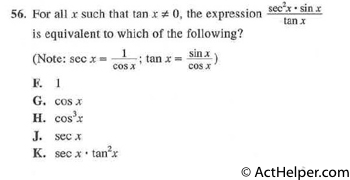
$$=\frac{\frac{1}{(\cos x)^{2}}\cdot\sin x}{\frac{\sin x}{\cos x}}$$ …
Choice E

Choice F
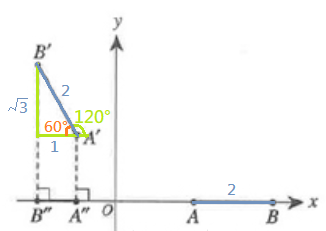
$$30°:60°:90°$$ $$=1:\sqrt{3}:2$$
Choice E
$$n\gt 0,$$ $$x,x+n,x+2n=120$$ $$\Rightarrow 3x+3n=120$$ …
Choice F
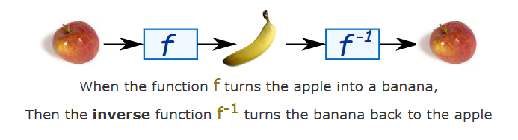
$$f(x)=\sqrt[3]{x+2}$$ $$=(x+2)^{-3}$$ $$f^{-1}(x)=x^{3}-2$$